I think I found a counterexample to the common wisdom that more walls always create a stronger part.
The pictured S shape is 1.5mm thick, so printing with 2 walls leaves no room for infill. My testing wasn’t very rigorous, but it seems that the hybrid structure of walls + rectilinear infill is 10-20% more rigid than walls alone. The infill adds strength by cris-crossing between adjacent layers.
I think it’s fine to include a concentric top/bottom layer, but multiple identical layers weaken the part. I also tried 0 walls (infill only) and that was garbage.
It’s like making a truss vs just straight pipes.
What testing did you complete? Curious to find out how you found it stronger. As in if this is done as a hook, can it hold more load ?
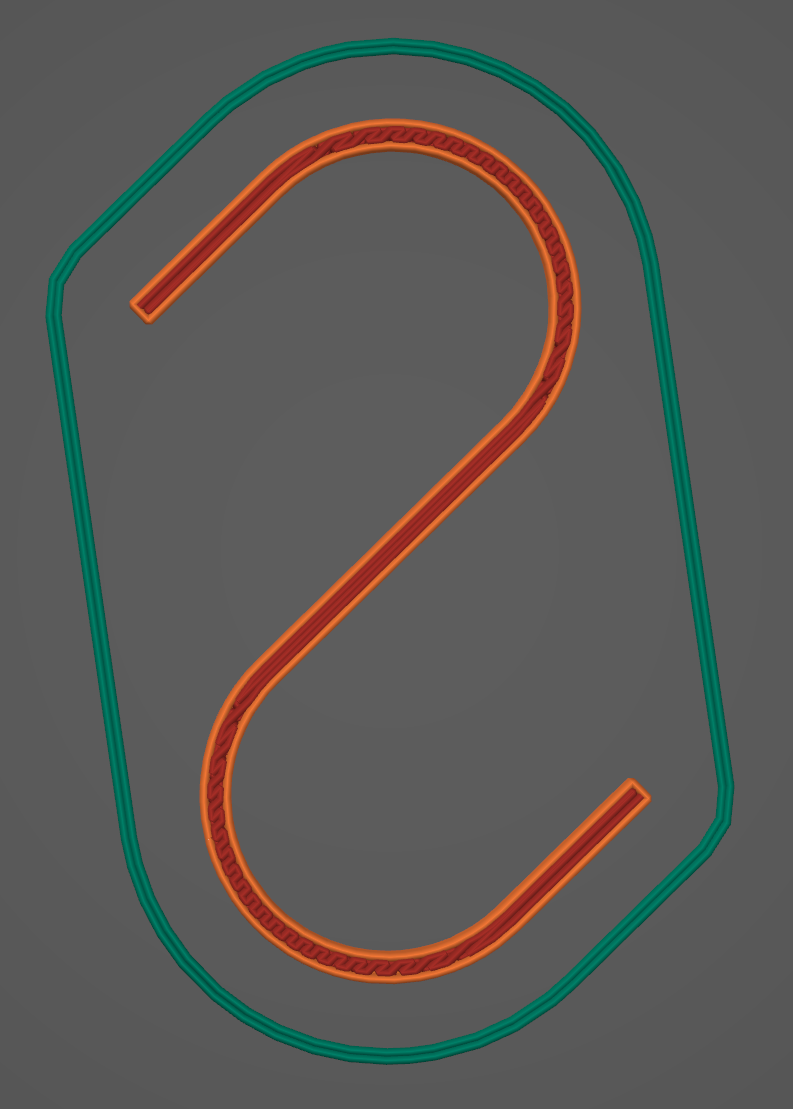
I just tested this hook by hanging a 5 gallon bucket and gradually adding water. PLA 1 wall = 1710 g, 2 wall = 1178 g. The thing is, a 1.5 mm hook fails by straightening rather than snapping, so rigidity is more important than strength.
Edit: I also tried PETG: 1 wall = 1441 g, 2wall <998 g (failed to hold the empty bucket)
So the 1 wall hooks can support >40% more weight before straightening enough that the bucket slips off. That’s more significant that I expected.
Edit2: Part of that 40% difference is due to friction; the 1-wall hook has a rough surface that makes the bucket less likely to slip off. But even with bluetack on the hooks, 1-wall has less deflection than 2-wall for the same weight.
Another confounding variable is the weight of the hook itself: 2-wall = (0.83g slicer, 0.78g actual); 1-wall = (0.95g slicer, 0.88g actual). I don’t know if that extra weight is coming from density or volume, but either way the stronger hook is more expensive.
Sigh, reality is so complicated.
Nice! Thanks for the work and reply 😊
Lock some calipers (with a rod sticking out) to 1.5mm shorter than the part height, compress it down onto a kitchen scale until the rod is just touching the platform, and record the weight.
That’s just the first procedure that came to mind. I will try to think of a way to do a hook strength test today.
A shape like an S is going to be highly anisotropic with regards to flexing and rigidity. Yes, more walls won’t really help if they are not in the direction to resist the force in question.
Like someone else mentioned, an I beam is basically as strong as a beam with the interior filled in, because that’s where the stress goes.
Shouldn’t that be obvious? The same way a bundle of hair is softer than a rod of the same thickness. The extreme of this are sandwiches like carbon - lightweight core - carbon. There, the parts that experience the biggest forces (the outermost layer in tension) is extremely stiff, while the core only needs to withstand compression to keep both apart.
No, that shouldn’t be obvious. First of all, the reason a bundle of hair is stronger and stiffer than a rod of equivalent area and material, is not necessarily applicable at the scale of 3D printing layers (there should be an effect, but it gets much more prevalent in smaller scales). Then second, your example with the CFRP Sandwich Panel is not really correct. A full thickness CFRP plate is going to be stiffer and stronger than a Sandwich panel. Will it be much heavier and much more expensive? Yes! Will it be stiffer and stronger? Yes, a little bit. Only specific properties (so, per kg or per dollar) are improved (and by significant amount). Also the core has to withstand out of plane shear in addition to compression and the bending stiffness is highly dependent on this shear stiffness. My assumption for OPs effect is, that the criss-cross pattern is stiffer than the interlaminar shear stiffness of the individual 3D printed layers (but thats just a guess, could also be a quality aspect). This is not true for CFRP Sandwiches. CFRP interlaminar shear stiffness is much higher than of the core material (at least in general). Sorry, don’t want to come across as an ass :)
Everything you say is correct and, to me, obvious and was never disputed.
I think the hair analogy fits this case, obviously it is not 100 % the same.
Really interesting! I wonder what would happen if you combine these two properties. Suppose some length of the middle is all walls, and the hooks are infill, or vice versa. Is there an optimal mix that maximizes the weight it can support in your testing, or have you found the optimal configuration (with infill along the entire length) already?
I haven’t done any tests where the tensile/breaking strength is relevant, just rigidity. Maybe it’s possible to optimize the infill based on finite element analysis or something, but that’s not a rabbit hole I’m looking to go down.
I have tested that an all-walls sandwich (PrusaSlicer “solid infill every 3 layers”) does not improve rigidity. So far nothing beats 1-wall + rectilinear in that department.